This is another one in the problem solving series. And it’s a little involved.
I have been running SAT (Scholastic Aptitude Test) coaching classes for some time now. And one of the things we do is give them mock tests once a week.
One time, two of the answer sheets had an unusually high number of answers that matched, including the ones that were wrong.
Here’s they are.

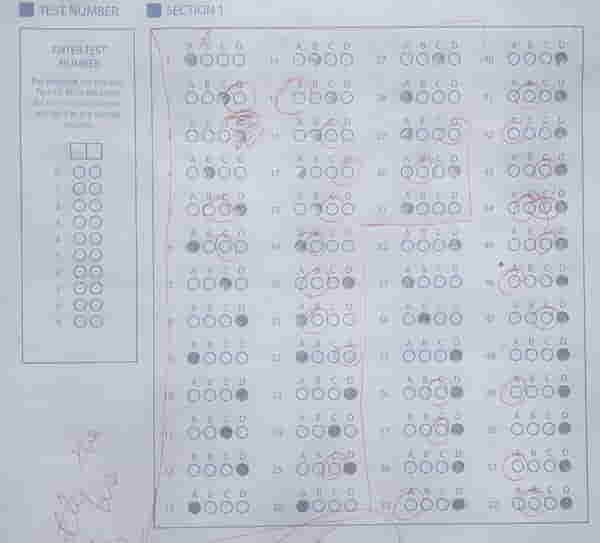
Look closely and you’ll notice that the first 31 answers the two students provided are exactly the same, including the wrong ones!
Assuming the two students did NOT cheat, what is the chance or probability that this could happen randomly? In other words, statistically, what’s the likelihood of that happening?
Again, like all the other problems of these kinds, it can be solved by modeling. If you know statistics, it can be solved quite readily using statistical formula too.
However, the similarities in answers sheets of these two students didn’t end or stop there. In the next mock test too, a number of answers were found to be the same, though not as many.
Here are the answer sheets.


As you can see from the images above, this time around, 13 of the first 31 answers are the same.
So, the next question is: what are the chances that both 31 in the first test AND 13 in the test that followed are the same?
(Incidentally, this is the seventh problem in the series of blog posts about challenging problems. Click here, here, here, here, here, and here for the first six. Click here for a description of the reason behind the series and what modeling means in this context.)
